The Witt vector affine Grassmannian
Apparaît également dans les collections : Arithmetic geometry, representation theory and applications / Géométrie arithmétique, théorie des représentations et applications, Fields medallists - 2018
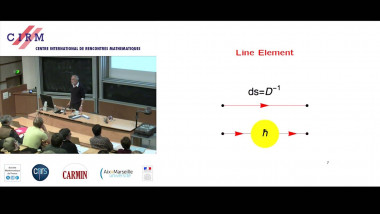
(joint with Bhargav Bhatt) We prove that the space of $W(k)$-lattices in $W(k)[1/p]^n$, for a perfect field $k$ of characteristic $p$, has a natural structure as an ind-(perfect scheme). This improves on recent results of Zhu by constructing a natural ample line bundle on the space of such lattices.