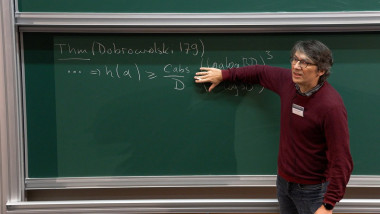De Tobias Mai
Apparaît dans la collection : Chaire Jean Morlet - Conference - Algebraic aspects of random matrices / Chaire Jean Morlet - Conference - Aspects algébriques des matrices aléatoires
Over the last couple of years, it has become evident that matrix-valued semicircular elements establish strong links between free probability theory and noncommutative algebra. Another surprising connection of this kind was found in a recently finished project with Roland Speicher. We have shown that the Fuglede-Kadison determinant of an arbitrary matrix-valued semicircular element is essentially given by the capacity of its associated covariance map. In addition, we have improved a lower bound by Garg, Gurvits, Oliveira, and Widgerson on this capacity, by making it dimension-independent. Besides analytic tools from operator-valued free probability, these are the crucial ingredients in some novel algorithmic solution to the noncommutative Edmonds' problem which we described in collaboration with Johannes Hoffmann. In my talk, I will present our work and provide the background on free probability and noncommutative algebra required for this purpose.
De Vladimir Dotsenko
De Arthemy Kiselev
