Apparaît dans la collection : Zeta Functions / Fonctions Zêta

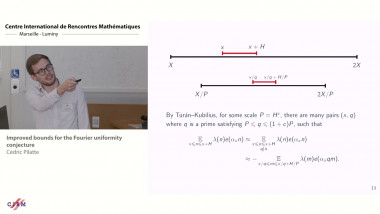
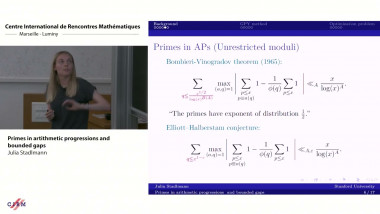
A number field is monogenic if its ring of integers is generated by a single element. It is conjectured that for any degree d > 2, the proportion of degree d number fields which are monogenic is 0. There are local obstructions that force this proportion to be < 100%, but beyond this very little is known. I’ll discuss work with Alpoge and Bhargava showing that a positive proportion of cubic fields (d = 3) have no local obstructions and yet are still not monogenic. This uses new results on ranks of Selmer groups of elliptic curves in twist families.