De Yuval Dor
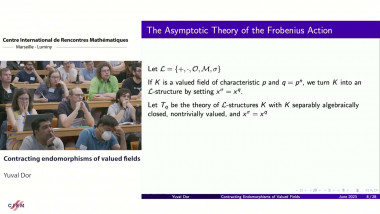
We propose a model complete theory of valued fields equipped with an $\omega$ increasing endomorphism which is not onto. The key to the quantifier elimination statement is a study of generic types and a systematic use of descent. As a consequence, we derive the decidability of the elementary theory of the Frobenius action on separably algebraically closed valued fields, generalizing results of Chatzidakis and Hrushovski. This is joint work with Yatir Halevi.
De Arthur Forey
De Sylvy Anscombe
De Raf Cluckers
De Pablo Cubides Kovacsics
De Lothar Sebastian Krapp
De Michal Szachniewicz
De Ya'acov Peterzil
De Mariana Vicaria
De Matthias Aschenbrenner
De Philip Dittmann
De Adele Padgett
De Barbara Schapira
De Barbara Schapira
De Alain Connes
De Teresa Krick
