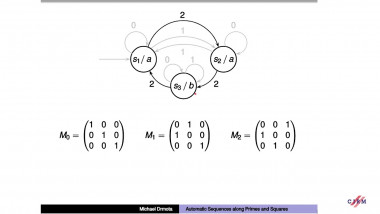
Zaremba's conjecture and growth in groups
Apparaît également dans la collection : Jean-Morlet Chair 2020 - Conference: Diophantine Problems, Determinism and Randomness / Chaire Jean-Morlet 2020 - Conférence : Problèmes diophantiens, déterminisme et aléatoire
Zaremba's conjecture belongs to the area of continued fractions. It predicts that for any given positive integer q there is a positive a, a < q, (a,q)=1 such that all partial quotients b_j in its continued fractions expansion a/q = 1/b_1+1/b_2 +... + 1/b_s are bounded by five. At the moment the question is widely open although the area has a rich history of works by Korobov, Hensley, Niederreiter, Bourgain and many others. We survey certain results concerning this hypothesis and show how growth in groups helps to solve different relaxations of Zaremba's conjecture. In particular, we show that a deeper hypothesis of Hensley concerning some Cantor-type set with the Hausdorff dimension >1/2 takes place for the so-called modular form of Zaremba's conjecture.