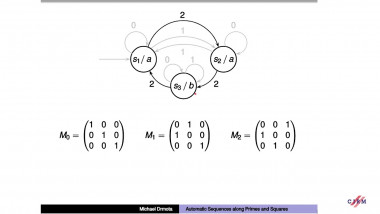
Normal and non-normal numbers
Apparaît également dans la collection : Jean-Morlet Chair 2020 (2) - Workshop: Discrepancy Theory and Applications - Part 2 / Chaire Jean-Morlet 2020 (2) - Workshop : Théorie de la discrépance et applications - Part 2
We fix a positive integer $q\geq 2$. Then every real number $x\in[0,1]$ admits a representation of the form
$x=\sum_{n\geq 1}\frac{a_{n}}{q^{n}}$,
where $a_{n}\in \mathcal{N} :=\{0,1,\ .\ .\ .\ ,\ q-1}$ for $n\geq 1$. For given $x\in[0,1], N\geq 1$, and $\mathrm{d}=d_{1}\ldots d_{k}\in \mathcal{N}^{k}$ we denote by $\Pi(x,\ \mathrm{d},\ N)$ the frequency of occurrences of the block $\mathrm{d}$ among the first $N$ digits of $x$, i.e.
$\Pi(x, \mathrm{d},N):=\frac{1}{N}|\{0\leq n< N:a_{n+1}=d_{1}, . . . a_{n+k}=d_{k}}$
from a probabilistic point of view we would expect that in a randomly chosen $x\in[0,1]$ each block $\mathrm{d}$ of $k$ digits occurs with the same frequency $q^{-k}$. In this respect we call a real $x\in[0,1]$ normal to base $q$ if $\Pi(x,\ \mathrm{d},\ N)=q^{-k}$ for each $k\geq 1$ and each $|\mathrm{d}|=k$. When Borel introduced this concept he could show that almost all (with respect to Lebesgue measure) reals are normal in all bases $q\geq 2$ simultaneously. However, still today all constructions of normal numbers have an artificial touch and we do not know whether given reals such as $\sqrt{2},$ log2, $e$ or $\pi$ are normal to a single base. On the other hand the set of non-normal numbers is large from a topological point of view. We say that a typical element (in the sense of Baire) $x\in[0,1]$ has property $P$ if the set $S :=${$x\in[0,1]:x$ has property $P$} is residual - meaning the countable intersection of dense sets. The set of non-normal numbers is residual. In the present talk we will consider the construction of sets of normal and non-normal numbers with respect to recent results on absolutely normal and extremely non-normal numbers.