Dispersive Integrable Equations: Pathfinders in Infinite-Dimensional Hamiltonian Systems / Équations Intégrables Dispersives, Pionniers des Systèmes Hamiltoniens en Dimension Infinie

Collection Dispersive Integrable Equations: Pathfinders in Infinite-Dimensional Hamiltonian Systems / Équations Intégrables Dispersives, Pionniers des Systèmes Hamiltoniens en Dimension Infinie

Organisateur(s) Gérard, Patrick ; Grava, Tamara ; Miller, Peter ; Visan, Monica

Date(s) 28/04/2025 - 02/05/2025

URL associée https://conferences.cirm-math.fr/3209.html

00:00:00 / 00:00:00

2 5

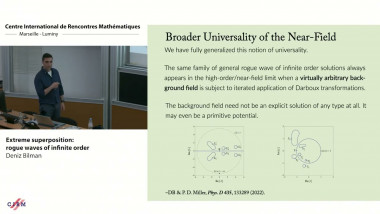
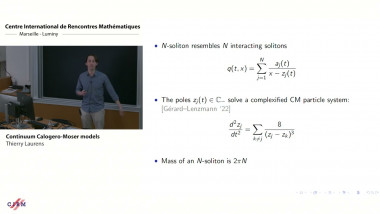
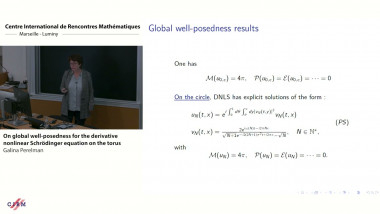
Continuum Calogero–Moser models

De Thierry Laurens

The focusing Continuum Calogero–Moser (CCM) equation is a completely integrable PDE that describes a continuum limit of a particle gas interacting pairwise through an inverse square potential. This system is well-posed in the scaling-critical space L2 below the mass of the soliton, but above this threshold there are solutions that blow up in finite time. In this talk, we will discuss some new and existing results about solutions below the soliton mass threshold. This is based on joint work with Rowan Killip and Monica Visan.

Informations sur la vidéo

Date de captation 28/04/2025
Date de publication 13/05/2023
Institut CIRM
Licence CC BY NC ND
Langue Anglais
Audience Chercheurs, Doctorants
Réalisateur(s) Guillaume Hennenfent
Format MP4

Données de citation

DOI 10.24350/CIRM.V.20344403
Citer cette vidéo Laurens, Thierry (28/04/2025). Continuum Calogero–Moser models. CIRM. Audiovisual resource. DOI: 10.24350/CIRM.V.20344403
URL https://dx.doi.org/10.24350/CIRM.V.20344403

Domaine(s)

Equations aux dérivées partielles

Bibliographie

KILLIP, Rowan, LAURENS, Thierry, et VISAN, Monica. Scaling-critical well-posedness for continuum Calogero-Moser models. arXiv preprint arXiv:2311.12334, 2023. - https://doi.org/10.48550/arXiv.2311.12334

Codes MSC

Document(s)

https://www.cirm-math.fr/RepOrga/3209/Slides/LAURENS.pdf

Dernières questions liées sur MathOverflow

Pour poser une question, votre compte Carmin.tv doit être connecté à mathoverflow

Poser une question sur MathOverflow

Toutes les vidéos de la collection

01:00:49

publiée le 13 mai 2023

Extreme superposition: rogue waves of infinite order

De Deniz Bilman

47:14

publiée le 13 mai 2023

Continuum Calogero–Moser models

De Thierry Laurens

58:32

publiée le 13 mai 2023

On global well-posedness for the periodic derivative nonlinear Schrödinger equation on the torus

De Galina Perelman

45:24

publiée le 13 mai 2023

Long-time behavior for the Benjamin-Ono equation: an example

De Louise Gassot

55:48

publiée le 13 mai 2023

Global well posedness and soliton resolution for the half-wave maps equation with rational data

De Enno Lenzmann

Copyright Carmin.tv 2025

Donner son avis