
Degenerations in convex projective geometry and non-archimedean ordered fields
De Anne Parreau
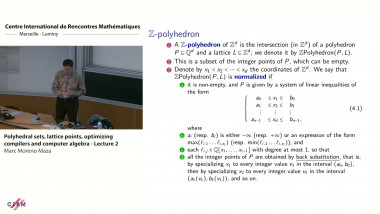
Polyhedral sets, lattice points, optimizing compilers and computer algebra - lecture 2
De Marc Moreno Maza

Polyhedral sets, lattice points, optimizing compilers and computer algebra - lecture 1
De Marc Moreno Maza












