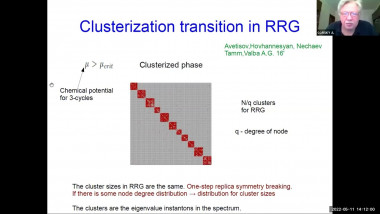
Spectral Flow Construction of 𝑁=2 Superconformal Orbifolds
Ten-dimensional Superstring theory unifies the Standard Model and quantum gravity. To obtain a four-dimensional theory with Space-Time Supersymmetry (which is necessary for phenomenological reasons), as shown by Candelas, Horowitz, Strominger, Witten, we must compactify six of the ten dimensions on a so-called Calabi-Yau manifold. Another equivalent approach to do the same is the compactification of 6 dimensions into an 𝑁=2 Superconformal field theory with the central charge 𝑐=9, as was shown by D. Gepner. Each of these two equivalent approaches has its own merits. In particular, Gepner's approach makes it possible to use exactly solvable N=2 SCFT models and thus obtain an explicit solution of the considered model. The subject of my talk is a new approach to the construction of Calabi-Yau orbifolds of Fermat type required for the compactification in Superstring theory. The idea of the approach is to use the connection of the CY orbifolds with a class of exactly solvable N=2 SCFT models for explicitly constructing a complete set of fields in these orbifold models using the Spectral flow twist (Schwimmer and Seiberg) and the requirement of the mutual locality of the fields.